(Not) Game Genres, pt. 12: Comparing Visuospatial Configurations and Terminology

Taxonomy of Virtual Spaces

Over the past several posts in my (Not) Game Genres series, I've reviewed numerous systems for analyzing video game space in comparison to my Taxonomy of Virtual Spaces system. This post serves as an overview of the Taxonomy and a summary of the previous posts together in one place.

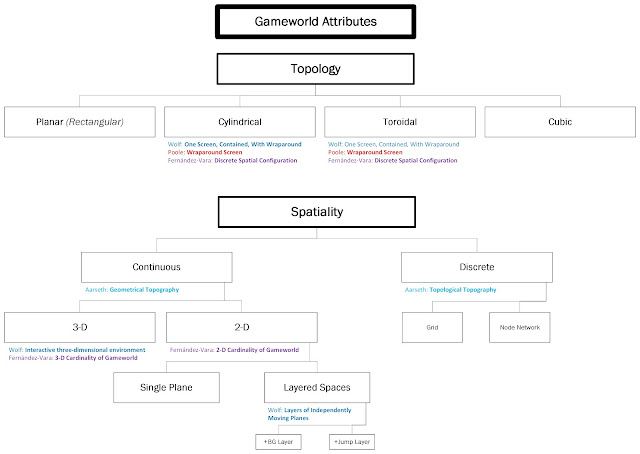
Each chart below presents the 

Mark J. P. Wolf's Elementary Spatial Structures of Video Games first published in "Inventing Space: Toward a Taxonomy of On- and Off-Screen Space in Video Games" from Film Quarterly (Fall 1997, vol. 51, no. 1). [previous post part 1 and part 2]

Steven Poole's Construction of Space in Video Games from Trigger Happy: The Inner Life of Video Games (2000). [previous post]

Espen Aarseth and his team's Multi-Dimensional Typology of Games from "A Multi-Dimensional Typology of Games" (in DiGRA '03 - Proceedings of the 2003 DiGRA International Conference: Level Up, 2003). [prevous post]

Clara Fernández-Vara and her team's Ontology of Spatial Configurations from "Evolution of Spatial Configurations In Videogames" (in DiGRA '05 - Proceedings of the 2005 DiGRA International Conference: Changing Views: Worlds in Play, 2005). [previous post]

Dominic Arsenault and his team's Game FAVR from "Game FAVR: A Framework for the Analysis of Visual Representation in Video Games" (in Loading… Journal of the Canadian Game Studies Association, vol. 9, no. 14, 2015). [no individual post, but discussed here and here]

The Game FAVR system is designed to analyze game visuals, and explicitly does not concern itself with game spatiality. Even so, I've adapted the core structure of Game FAVR (especially with the Three Image Planes) as the basis of my own system to analyze the visuospatial configuration of a virtual environment.

Gameworld

The gameworld is the defining qualities of a game’s virtual environment, as defined here by its topology and spatiality. A game may, and often does, feature several gameworlds divided into separate “worlds,” “levels,” or “campaigns,” depending on the game’s terminology.

The Topology of a gameworld deals with how the game's world is mapped out and what happens when one reaches the edge of the world. There are several single-screen "wraparound" games, most notably Pac-Man (Cylindrical) and Asteroids (Toroidal). Additionally, some smoth-scrolling games use these types of topologies, including Defender (Cylindrical) and Bosconian (Toroidal). These topologies aren't just for 2-D games, either. Hideo Kojima and Guillermo del Toro's horror game demo P.T. also features a Cylindrical topology where the player's path is on a loop. Other spatial systems lump these different topologies under the "wraparound" concept, though Poole does note that games like Asteroids are Toroidal.

As yet, the only game I've found that uses Cubic topology is E.T. for the Atari 2600.

To me, the spatial structure of the gameworld is expressed through the player's affordances in navigating that world, what Clara Fernández-Vara and her team call the "Cardinality of Gameworld." A game's visuals (what Clara Fernández-Vara would call "Spatial Representation") may appear three-dimensional, but a player's movement may only be restricted to a two-dimensional plane, or only a limited grid of discrete spaces.

The Continuous/Discrete Spatiality dichotomy is the only point where Aarseth's system intersects with my own.

This miscellaneous category expresses additional details about how the player understands the gameworld, such as the presence of a Mini-Map or Non-Euclidean Geometry. Gameworlds are also classified by presence and type of Gravity, though this quality has more affect on the player's ability to navigate in a game rather than giving a sense of spatiality. I will probably move this aspect into the player's affordances.

Wolf is the only other author to note that "mapped" spaces (mini-maps) are important to a player's sense of a game's space. A mini-map provides information about what is happening off-screen, allowing the player to think about an entire environment as a whole rather than just what is in front of their eyes. Wolf also makes mention of non-Euclidian geometry, without specifically using that term.

Framing Device

The frame, or the game screen, is the player’s window on the game world. This defines how the frame pans across or trucks through the environment and what directional controls the player has over the frame. 

The frame's mobility across the gameworld deals with what Clara Fernández-Vara and her team call the "Spatial Configuration." The directions the frame may move across the gameworld is what they call the "Cardinality of Gameplay." These are both different from the "Spatial Representation" (if the game looks like it has 2-D or 3-D objects) and "Cardinality of Gameworld" (the axes along which a player avatar may move through the gameworld). Personally, I find the terms Cardinality of Gameplay (defined as "how the player can move around the gameworld") and Cardinality of Gameworld (defined as "the way in which the player can navigate the space") unclear, as the terms "move" and "navigate" are not explicitly defined and differentiated.

Image Planes

Similar to the guidelines of the Game FAVR, each game image is divided into the conceptual planes of Agents, Environment, and Background/Foreground.

The agents are any interactive characters or objects in the game, including the player avatar and projectiles. The environment is the tangible space where agents navigate through and around. Background/foreground was chosen as more commonly understood terminology to replace Game FAVR's “off-game environment.”

Projection methods are formal, detailed qualities about how the objects in the image plane are “drawn” to the screen. This is close to what Clara Fernández-Vara and her team refer to as "Spatial Representation." The Game FAVR deals with formal qualities of game visuals and my system closely mirrors it. I adapted detailed, standardized terminology used in computer graphics from  "Planar Geometric Projections and Viewing Transformations" by Ingrid Carlbom and Joseph Paciorek (in Computing Surveys, vol. 10, no. 4, 1978).

The apparent projection angle is either Dynamic or fixed at a specific angle above the horizon line. Each fixed projection is measured or estimated for the frame and rounded to the nearest 15° increment between 0° (horizontal view) and 90° (overhead view) (see Figure 8). From the initial qualitative research performed, many games use , 90°, 35° (the standard dimetric (or "pixel isometric") projection angle), 15°, and 0°. The Game FAVR is the only other system that deals with projection angles.

Conclusion

Other systems analyzed here focus solely on the spatiality of digital games. The Game FAVR explicitly serves as a tool for analyzing the graphic presentation of digital games. My Topology of Virtual Spaces is designed as a tool for analyzing the visuospatial qualities of digital games and, unsurprisingly, uses many non-overlapping details of the above systems to achieve this goal.

(Not) Game Genres, pt. 11: Clara Fernández-Vara's Evolution of Spatial Configurations in Videogames

Evolution of Spatial Configurations In Videogames

The following text is adapted from my paper, "A Taxonomy of Virtual Spaces" (Rowe, unpublished):

Clara Fernández-Vara and her team at Georgia Tech (José Pablo Zagal and Michael Mateas) took issues with Mark J. P. Wolf’s Elementary Spatial Structures of Video Games ("Inventing Space: Toward a Taxonomy of On- and Off-Screen Space in Video Games," Film Quarterly, vol. 51, no. 1, 1997) (as described in part 6 and part 7 of this series of blog posts) when they developed their definitions of spatial properties of digital environments. They felt that, “his analysis lacks a historical perspective, and the strict comparison to film misses what the intrinsic properties of the digital medium bring to videogames” (Fernández-Vara, Zagal & Mateas, "Evolution of Spatial Configurations In Videogames," Paper presented at the DiGRA '05 - Proceedings of the 2005 DiGRA International Conference: Changing Views: Worlds in Play, 2005). The team worked on their own vocabulary for defining the spatial configurations of video games as part of the Game Ontology Project that had just started. The team focused only on defining how computers generate visual spaces procedurally, so they explicitly excluded any games that import spaces from other media (such as text adventure and full motion video games).

Their analysis focused on the relationship between the game space and the screen: “The screen is the basic unit of space in videogames, since it frames the interface” (Fernández-Vara et al., 2005). The screen is more than a window onto the digital world, it is also the yardstick by which the entire space (which they dub the “gameworld”) may be measured. A gameworld that extends beyond the limits of a single screen is segmented into screen-sized fragments.

The team also focuses on defining game features by their cardinalities: the spatial axes those features are restricted to. Gameplay is defined by the cardinality of player movement. Spatiality is defined by the cardinality of the gameworld. Separately from both cardinalities, the spatial representation may be in 2-D or 3-D. Finally, how the screen frame moves across the gameworld may be defined as single-screen (entire gameworld shown on one screen), discrete (entire screen refreshes to show a new location), or continuous (smooth-scrolling).

Ontology of Spatial Configurations in Videogames, as presented in "A Taxonomy of Virtual Spaces" (Rowe, unpublished). Note that the "(includes wraparound)" note for Continuous Spatial Configuration should instead by added to Discrete Spatial Configuration.

This Ontology of Spatial Configurations (my term, not the Georgia Tech team's) has been an influence in my own work, especially the concept that the screen is the "basic unit of space" in digital games (which I posted about previously). My own approach differs on several counts, as shown in the following comparison of the Ontology with my own Taxonomy of Virtual Spaces.

Spatial Representation

This refers to the whether the game's world is presented as either a 2-D or 3-D environment. This does not take into account how the player navigates through said environment, which falls under Cardinality of Gameworld. The Ontology includes isometric projections (referred to as "perspective" in the Ontology) in the 3-D category, as they present the appearance of three-dimensional forms.

My theory posits that a sense of spatiality is strongly related to both a player's affordances for navigation in a virtual space and the visuo-spatial configuration of that space. Together, these aspects define the spatial paradigm that may be used as a guide for the knowledge and techniques for creating games of a specific artistic style.

In my Taxonomy, the concept of Spatial Representation is taken up by the Projection Methods used for the various Image Planes that make up an image (the conceptual Agents, Environment, and Background/Foreground planes). This system is heavily based on the Game FAVR system developed by Dominic Arsenault and his team at the University of Montreal (Arsenault, Côté, & Larochelle, "Game FAVR: A Framework for the Analysis of Visual Representation in Video Games," Loading… Journal of the Canadian Game Studies Association, vol. 9, no. 14, 2015) and goes far into details beyond 2-D or 3-D Spatial Representation.

Cardinality of Gameworld

This refers to the dimensions along which the player avatar may navigate through a game's space. This may or may not match with the dimensions of Spatial Representation.

My Taxonomy mostly focuses on how a player avatar is able to navigate through a virtual space, For example, Zaxxon presents a 3-D Game Space that the player flies through, but Q*bert's pyramid of cubes is really a 2-D, Triangular Grid of Discrete Game Spaces, with the unusual background layer a character may jump into to meet their doom.

Cardinality of Gameworld in the Ontology matches closely with my categories of 2-D and 3-D Continuous Spatiality in my Taxonomy. The Taxonomy further accounts for layered 2-D spaces and node networks of discrete spaces (such as in chess and the afore-mentioned Q*bert).

Spatial Configuration

This aspect strictly with the screen as a framing device on the gameworld. The Georgia Tech team defines this as "the dichotomy between discrete and continuous spaces."

This dichotomy considers how the virtual space is contained within [the] frame, whether the gameworld is encompassed within a single screen, or extends beyond its limits. In the second case, the representation must be segmented, and the player will experience that space in a fragmented way.

This segmentation can be realized either in a discrete or a continuous way. Discrete segmentation occurs when the screen contains one fragment of the gameworld, which the player navigates; when she reaches the limits of that fragment, the screen refreshes to a different segment of that space... This segmentation may also affect the gameworld, e.g. the player character can move from one segment to another, but the enemies will not follow the character to the next segment (e.g. Prince of Persia, PC, 1989). On the other hand, the space is represented continuously when the screen is showing with a scroll... or moving the point of view of the player as she moves around. (Fernández-Vara et al., 2005)

This game screen is what my Taxonomy calls the Framing Device and the closest thing to Spatial Configuration is Frame Mobility, which is loosely influenced by this very Ontology and by the Game FAVR system developed by Dominic Arsenault and his team at the University of Montreal (Arsenault et al., 2015).

"Single-Screen" spatial configuration is Fixed Frame.

"Discrete" spatial configuration is Discrete Frame Mobility. This is sometimes referred to as "page flip" scrolling, like in Pitfall or Adventure on the Atari 2600.

"Discrete" spatial configuration also includes "wraparound" single screens (there is an error in the Ontology of Spatial Configurations chart I copied from my document, above). These are what I define as Cylindrical or Toroidal Topologies.

"Continuous" spatial configuration is Smooth Scroll Frame Mobility.

"Locked Scrolling" is Auto-Scroll Frame Mobility. This is what the Game FAVR system refers to as "Authoritarian Framing Mobility."

Note the major difference here between the Georgia Tech team's concepts of discrete and continuous spaces and my Taxonomy's concepts of discrete and continuous spatiality, which I adapted from Noah Wardrip-Fruin's How Pac-Man Eats (2020) and wrote about explicitly at the end of my previous post in this series. 

Cardinality of Gameplay

This section deals with the axes along which the player is able to move the frame across the gameworld. This specifically excludes single-screen games, as the frame in that case is fixed and the entirety of the gameworld is presented on the screen.

One-Dimensional Gameplay is where the player may move through the world along a single axis, such as in side-scrollers and vertical shooters. This is akin to how I define the 2-D Frame Mobility Direction by the axis along which the frame may move (Horizontal Axis Only, Vertical Axis Only, or Diagonal Axis Only (like Zaxxon) and how many directions the player may move along that axis (One Direction or Two Directions).

Two-Dimensional Gameplay allows for scrolling along both the X and Y axes. This is equal to my 2-D Frame Mobility Direction definitions of Horizontal and Vertical Axis or Any Direction. Additionally, I have a category of Horizontal or Vertical Axis to account for the peculiarities of some Nintendo NES/Famicom games (such as Metroid).

Three-Dimensional Gameplay is accounted for in my 3-D Frame Mobility Direction classifications of moving the camera by Yaw, Pitch, Roll, and Into the Z-Axis.

Conclusion

The Georgia Tech team's Ontology of Spatial Configuration was a strong influence on my own work, although their Ontology and my Taxonomy are notably different. By their own words, the Georgia Tech team set out to define "basic spatial configurations" using "a few basic features" (Fernández-Vara et al., 2005). Conversely, my system is intended as a formal system to inform production and detailed analyses of digital games as aesthetic objects.

(Not) Game Genres, pt. 10: Espen Aarseth's Multi-Dimensional Typology of Games

Espen Aarseth is an academic who has long been an evangelist for the importance of the concept of virtual spatiality to understanding digital games. I've posted about Aarseth previously in regards to digital games as texts. Aarseth is well-known in game studies for the concept of "ergodicity" in games.

Aarseth tackled the subject in question in "Allegories of Space: The Question of Spatiality in Computer Games" (in Cybertext Yearbook 2000, edited by M. Eskelinen and R. Koskimaa, Jyväskylä Finland: University of Jyväskylä, 2001). In it, he argues that, "the problem of spatial representation is of key importance to the genre’s aesthetics," and that spatiality is, "the defining element in computer games." He analyzed the evolution of digital games with regard to spatiality and concluded that computer games could be classified by how they implement spatial representation. At the end of the article, he lamented that he could not do the task himself, so "a thorough classification… would need much more detailed analysis than there is room for in this study."

A Multi-Dimensional Typology of Games

In 2003, Aarseth partnered with Solveig Marie Smedstad and Lise Sunnanå to write "A Multi-Dimensional Typology of Games" (in DiGRA '03 - Proceedings of the 2003 DiGRA International Conference: Level Up, Utrecht The Netherlands: University of Utrecht, 2003), presented at the first DiGRA conference. As quoted from the paper, this is an attempt to codify a model for classifying different "games in virtual environments." Aarseth et al classify games across 15 "dimensions" that are grouped under five different headings: Space, Time, Player-structure, Control, and Rules.

For my analysis, I focus on the heading of Space, which the paper describes as follows:

Space is a key meta-category of games. Almost all games utilize space and spatial representation in some way, and there are many possible spatial categories we could use, a typical one being the distinction between 2D and 3D games. However, this distinction seems to be mostly historical, since the early games were mostly 2D and the modern games are usually 3D. Also, it does not allow for a good representation of board games, which are two-dimensional in movement, but three-dimensional in representation. This problem holds for many computer games as well.

From this description, the Multi-Dimensional Typology specifically does not deal with the dimensionality of the space presented on the screen (2-D or 3-D). The paper derides the distinction as "mostly historical," with modern games tending to fall into the 3-D category. With the rise of indie games, nostalgic interest in older game styles and aesthetics, and the emergence of new game platforms (such as smartphones), 2-D games have remained in vogue in the years since this paper was published. 2-D spatiality is now a tool for game developers to use in order to best present their creation, rather than a concession to technological limitations.

Aarseth's &co's define three dimensions under the heading of Space: Perspective, Topography, and Environment. Each game may be classified by where it falls on each of these conceptual dimensional axes. 

Perspective: Omni-present, Vagrant

The perspective is considered omni-present if the player is free to view the entirety of the game space at will, like in many strategy games. Some of the game may be blocked by "fog of war," for example, but the player may still move around, typically as a disembodied camera.

The perspective is considered vagrant is the player's view is restricted to following a game avatar. These games may be classified by their visual perspectives as either 1st person or 3rd person.

Aarseth's "perspective" dimension deals with the ocularization of the game - who's eyes do we see the game through? Dominic Arsenault and his team at the University of Montreal include this concept of ocularization in their Game FAVR analysis system ("Game FAVR: A Framework for the Analysis of Visual Representation in Video Games," Arsenault, Côté, & Larochelle, 2015), based on the works of François Jost ("Narration(s): En deçà et au-delà," Communications, 38, p. 192-212, 1983) and Stam, Burgoyne, & Flitterman-Lewis (New Vocabularies in Film Semiotics: Structuralism, Post-Structuralism, and Beyond, London/New York: Routledge, 1992) in creating tools for studying storytelling in films. Arsenault et al modified the methods to account for digital game scenes like menu screens that do not appear in films.

My typology deals with the construction of virtual spaces and navigation through those spaces, not with ocularization.

Topography: Geometrical, Topological - 

A game with continuous freedom of movement is geometrical. The example of Quake Arena allows for player movements "in all directions, with millions of alternative positions, and the player's position in the game-world can be moved one miniscule increment at a time." This is what I call Continuous Spatiality in my own taxonomy.

A game with discrete, non-overlapping positions to move between is topological. The example given in chess, where only one piece may occupy any of the 64 discrete squares on the chessboard. This is what I call Discrete Spatiality that may be further categorized into Grid or Node Network. A chessboard is an example of a grid.

Environment: Dynamic, Static - 

A dynamic environment is one where the player can manipulate and modify it during gameplay (such as constructing bridges and digging in the dirt in the game Lemmings).

A static environment cannot be changed by the player. A player opening and closing doors in an environment merely changes the status of those doors, thus the environment would still be considered static. Similarly, environments where the player may build buildings (Warcraft or Age of Empires) without meaningfully changing the environment still count as static.

My typology deals with the construction of a spatial phenomenon experienced by the player, not the ability to change an environment. This dimension does not match anything in my system.

Figure 1 from "A Multi-Dimensional Typology of Games" showing titles organized along three dimensions of Perspective, Topography, and Environment

The other Multi-Dimensional Typology dimensions deal with other aspects of games, such as how time flows, number of players, adversaries, and the ability of the player to save their progress.

The dimensions under the Space heading and my Taxonomy of Virtual Dimensions do not have any overlap except for the concepts of Continuous and Discrete Spatiality. I describe the differences between the two as follows in my paper, A Taxonomy of Virtual Dimensions (Rowe, unpublished):

Two of the earliest contenders for the title of “first video game” are Christopher Strachey’s Draughts (1951) (Figure 4) and Willy Higinbotham’s Tennis for Two (1958). Each title is pioneering in its own right: Draughts is probably the first game a computer game program and the first computer game with graphics on a cathode ray tube while Tennis for Two is the first known two-player action game. Analyzing these two games for their presentation of spatiality would help us articulate what is important about these two works.

Draughts has what Noah Wardrip-Fruin would describe as Discrete Spatiality. The entire game space is “divided into non-overlapping spaces, and each game action involved moving a piece from one discrete space to another with no in-between position available or meaningful” (Wardrip-Fruin, How Pac-Man Eats, 2020). Each square on the checkerboard is a separate point in space. The checkers do not move between the points as there is no “space” to move through. Many strategy games work in this same manner today. Sprites may animate as if they are moving between spaces, but the game only treats them as being in one space or another, never overlapping multiple spaces.

Conversely, Tennis for Two is the first example of Continuous Spatiality in a digital game, which “requires that there be many potential positions in the virtual space (so many that moving between them creates a feeling of continuousness)” (Wardrip-Fruin, 2020). It is also worth noting that time in the game is discrete (time tracked by alternating game turns of any length) or continuous in each example.

(Not) Game Genres, pt. 9: Steven Poole's Construction of Space in Video Games

In my previous chapter in this series about game spatiality, not game genres, I reviewed Nicholas Caldwell's paper, Theoretical Frameworks for Analysing Turn-Based Computer Strategy Games. Caldwell based his methodology for analyzing strategy games on Steven Poole's use of Charles Peirce's typology of signs as found in Poole's book, Trigger Happy (2000).

Trigger Happy: The Inner Life of Video Games

Trigger Happy (Poole, 2000, Arcade Publishing)

Trigger Happy was an early text that took a serious look at digital game history and made attempts to analyze the aesthetics of such games, comparing them to techniques established in painting and cinematic arts. This was an era when academia all but ignored video games, one year before Espen Aarseth co-founded the journal Game Studies, back when we still referred to digital media as "new" media. Thus, the task of penning this book fell to a journalist, not an academic. Steven Poole has written articles for The Guardian, Edge, The Telegraph, and other publications, along with a number of other books. Since Trigger Happy's publication, it has been cited many times in refereed journal articles and books.

[References to page numbers in the analysis below refer to the "2007 web download edition" that Poole freely released on his website for a limited time and is under the CC BY-NC-ND 3.0 license. It can now be accessed on archive.org.]

Digital Games as Art

Aside from the aforementioned typology of signs analysis work, Poole's work corresponds with my research in a number of aspects, including the need for a language to speak to the unique aesthetic qualities of digital games: "Videogames are an increasingly pervasive part of the modern cultural landscape, but we have no way of speaking critically about them" (pg. 30). Video games were not generally accepted as a serious form of art. Then again, neither were films or jazz music in the early 20th century, yet now they are generally accepted as important aesthetic forms of human expression worth of critical study and analysis. Poole predicts that digital games will hold the same appreciation by the middle of the 21st century (pg. 32). Hopefully, we are well on our way to beat that timeline.

Game designer Chris Crawford similarly stated that "computer games constitute a new and as yet poorly developed art form" over a decade earlier in The Art of Computer Game Design (1984, Osborne/McGraw-Hill). This pioneering book is now regarded as the first full publication devoted to what would later be named game studies (Wolf and Perron, The Video Game Theory Reader, 2003, Routledge). Crawford stated that we need to establish "a framework for criticism" as part of our "path to understanding" digital games as an art form (1984). Since Crawford's and Poole's books were published, game studies scholars have adapted "frameworks for criticism" from "new art history" methods used in Marxist, feminist, and postcolonial theories, for example.

I argue that the last missing element for Crawford's "path of understanding" is "our principles of aesthetics" (1984). We have the structure to critique digital games, but still lack an agreed-upon language for discussing digital games as an art unto themselves and "speaking critically about them" (Poole, 2000).

Poole cites a French text, L'univers des jeux vidéo (Alain and Frédéric Le Diberder, 1998, la Découverte), which includes digital games as the "tenth art." The six classical arts are music, poetry, architecture, painting, dance, and sculpture. The Le Diberders add new arts for the 20th century: television, films, graphic novels, and digital games (Poole, pg. 29).

The "ten arts" concept reminds me of Henry Jenkins' declaration that digital games are one of the "lively arts" (Jenkins, "Games, the New Lively Art" In Handbook for Video Game Studies, 2005, MIT Press, pp 175-189). Jenkins refers back to Gilbert Seldes and his early critical text on pop culture, The Seven Lively Arts (1923, Sagmore Press). Seldes defends "lowbrow" entertainments that he terms "lively" arts (including slapstick comedies, musical theater, popular music, vaudeville, and comic strips) against the seven major "hibrow" arts. At that time, the classical six arts referenced above had been updated to seven modern arts: painting, sculpture, literature, architecture, theater, film, and music. Jenkins declares that digital games are a new lively art, "one as appropriate for the digital age as those earlier media were for the machine age" (Jenkins, pg. 177).

Art History of Games?

"Videogames have... repeated histories of representation in art, on jittery, caffeine-fueled fastforward. But it is immediately apparent that so far, they have only reached a surprisingly early stage in that development, for by the eighteenth century in painting the classical ideal of beauty based on some cosmic mathematical order was already being challenged, and the shortcomings of perspective were already being identified. Videogame scenery, being an artifact of computers, is clearly still in thrall to the god of mathematics. Of the myriad post-perspectival ways of seeing such as impressionism or cubism, there is as yet no sign in the apprentice draughtsmanship of videogames" (Poole, 2000, pp 236-237).

I often feel that AAA studios seem like the French academic painters of the early 1800s, highly skilled at rendering ever-greater levels of visual fidelity, delicate interplay of light, and beautiful forms. Digital games only slowly creep toward what might be considered our versions of the modern art styles. Occasionally, a Grim Fandango or a Kentucky Route Zero will come along, paying tribute to one or more of these "modern" styles of last century. Rarely does a game attempt a sort of space-bending reality commonly seen in cubist, surrealist, Dadaist, expressionist, and other modern styles.

Digital Game Visuo-spatiality

Trigger Happy also includes a "brief history of the construction of space in video games" (pg. 236) in chapter 6: "Solid Geometry" (pp 199-239). Poole looks at the touchstone moments in stylistic presentations of space that bring developers closer to the "Holy Grail" of game environments: "a 'virtual' space that the player could inhabit" (pg. 204).

These stylistic innovations were afforded by means of technological innovations and advances in display and computing hardware. However, Poole states, "It is important to emphasize... that innovations... did not at once render earlier forms obsolete" (pg. 212). Innovations added more tools to the game developer's toolbox rather than just replacing the old tools. As an example, scrolling screens did not eliminate the need for fixed-screen games. Robotron 2084 (1982) works so well as a frantic, exciting game precisely because the player is trapped on one screen, in a claustrophobic space where there is nowhere to run. An interview with Robotron creator Eugene Jarvis confirms this was a intentional, aesthetic choice:

"With Robotron, I just stuck the guy on one screen... There's two hundred robots trying to mutilate you, and there's no place to hide... It was an incredible sweaty palms experience. It's just confinement. You are stuck in that room. You can't run down the hallway. You can't go anywhere else. You're just totally focused. A lot of times, the games are about the limitations. Not only what you can do but what you can't do. Confining your world and focusing someone in that reality is important." Quoted from Joystick Nation by J. C. Herz (1997, pp 78-79)

Poole intentionally likens the history of the projection of virtual spaces to innovations that developed in art history, especially with painting but also in cinema, "Videogames have rehearsed other histories of pictorial representation and come up with imaginative and original visual strategies themselves" (pg. 206). 

The following is an analysis of Poole's terminology (including some terms from Poole's pseudo-sequel, Trigger Happy 2.0 (2013), as compared to my own Taxonomy of Virtual Spaces. Poole did not name each type of projection method, sometimes merely describing This is similar to my analysis of Mark J. P. Wolf's "Elementary Spatial Structures of Video Games" (1997, 2001) that I posted previously (part one, part two).

1. No environment - "In the earliest videogames... the environment had no characteristics of its own: it was not terrain, but simply a function of the relations between objects or a means by which time could pass while one object traveled across the screen, so that everything did not happen simultaneously" (Poole, pg. 207). I essentially describe this as no environment image plane, but Poole gives a compelling description for what is essentially nothing.
2. Fixed screen - "The boundaries of the TV screen limited the play arena to a fixed, small size, and thus limited the type of action available to game designers... The screen was a prison" (pg. 207). This is a fixed framing device with no mobility (in other words, the game "camera" doesn't move).
3. "Wraparound" screen - "Topologically, the spatial arrangement of Asteroids, though it looked flat, was actually equivalent to the surface of a torus (a doughnut with a hole in the middle)" (pg. 208). Just like Poole describes, I say this is a toroidal topology. Another form of "wraparound" screen may have cylindrical topology (like Pac-Man).
4. "Scrolling" screen - "The superficial limits of the screen were further eroded by the invention of scrolling" (pg. 208). Poole's example of Defender uses smooth scrolling framing device with horizontal mobility.
5. "Ineluctable scrolling" screen - "Unstoppable, ineluctable scrolling." "For reasons rarely explained by the developers of arcade games such as Scramble, your ship had a minimum speed below which it could not operate... moving forwards at a certain rate, towards your doom, was existentially obligatory... in sidescrollers and upscroller, the game scrolls regardless of your input. It's more like an enemy than a tool, even as it is also the engine of your apparent progress" (Poole, Trigger Happy 2.0, ebook, 2013, chapter 2). An auto-scrolling framing device.
6. "Parallax" scrolling - A multi-planar background image plane.
7. "Isometric perspective" - Regarding Zaxxon, "You could see three sides of an object rather than just one. And now, crucially, the game screen was not just a neutral arena - it had become an environment" (2000, pg. 214). For a time, "the most technologically sophisticated means of building a 3D world" (pg. 216). In comparison to "scientific perspective," "Foreshortening implies a subjective, individual viewpoint, so its absence in isometric graphics, along with the elevated position of survey, conspired to give the user a sense of playing God in these tiny universes" (pg. 216). This is dimetric projection environment plane and agent plane. "Isometric" has become a catch-all phrase for a number of related axonometric methods of projection (isometric, dimetric, and trimetric).
8. "Perspectival" - Games like Battlezone use "scientific perspective" (pg. 205). The Battlezone example uses 2-point perspectival projection environment plane and agent plane.

Coda?

"I will reinvigorate the retro game-reviewing vocabulary, since that will be the only way to distinguish oneself in an increasingly crowded media-critical space from the hordes of reviewers who are all now like Huizinga this and affordances that" (Poole, 2013).